
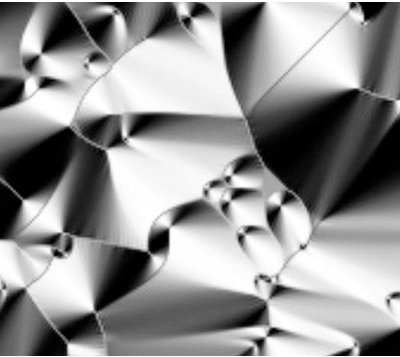
Computer simulation of the surface of a smectic liquid crystal, showing conical patterns like those seen under a microscope (image from the article “Weirdest martensite: smectic liquid crystal microstructure and Weyl-Poincaré invariance” published in Physical Review Letters)
The material looks like a mosaic of intersecting ellipses, parabolas or hyperbolas. Its structure, which has intrigued physicists for a century, has been revealed by means of Weyl-Poincaré invariance.
The material looks like a mosaic of intersecting ellipses, parabolas or hyperbolas. Its structure, which has intrigued physicists for a century, has been revealed by means of Weyl-Poincaré invariance.

Computer simulation of the surface of a smectic liquid crystal, showing conical patterns like those seen under a microscope (image from the article “Weirdest martensite: smectic liquid crystal microstructure and Weyl-Poincaré invariance” published in Physical Review Letters)
By José Tadeu Arantes | Agência FAPESP – Brazilian and US researchers have recently solved a riddle that has baffled physicists for a century. An article reporting the result, entitled “Weirdest martensite: smectic liquid crystal microstructure and Weyl-Poincaré invariance”, is featured on the cover of the April 8, 2016, issue of Physical Review Letters. Non-subscribers to the journal can read the full article at http://arxiv.org/pdf/1511.02252.pdf.
The group used computer simulations to describe the microstructures of smectic liquid crystals. In smectic phases, molecules are arranged in hundreds of evenly spaced layers only nanometers apart. The molecules in each layer can move about freely, just as in a liquid, but the layers in each region of the material are spatially ordered, as in concentric spheres. Different groups of layers may intersect, producing “defects” that often resemble segments of ellipses, parabolas or hyperbolas, curves that since antiquity have been called “conics” because they can be generated by the intersection of a cone and a plane.
Thus, when a smectic liquid crystal is confined between two slides and viewed under a microscope, it looks like a mosaic whose component parts are delimited by conic sections.
“These conic patterns have been studied for over a century, starting with groundbreaking work performed in 1910 by the French physicist and mineralogist Georges Friedel (1865-1933). He deduced that if a smectic liquid crystal formed such patterns when confined between glass slides under a microscope it must be made up of evenly spaced layers of molecules,” said Danilo Barbosa Liarte, first author of the article.
Liarte, currently working at Cornell University in the US, was awarded a postdoctoral scholarship by FAPESP for the research project “Statistical models for spin glasses and complex fluids”.
“The main challenge was understanding how space could be filled with these conics,” Liarte said. “We managed to solve the problem by making an analogy between the structures of smectic liquid crystals and the structure of martensite, a crystalline phase of steel.”
Martensite, named after the German metallurgist Adolf Martens (1850-1914), also has an unusual structure, combining regions with differing deformations and orientations. This gives it a hardness factor far superior to other forms of steel. However, smectic liquid crystals and martensites are entirely different materials. What they have in common are their microstructures, in which several different but compatible low-energy configurations coexist.
The conics that appear in smectic liquid crystals are called defects because they occur at places where a set of concentric molecular layers is interrupted, and the adjoining molecules located beyond the line are arranged in another set. A defect is an intersection between these two sets. Different sets constitute variants of a smectic liquid crystal.
“By analogy with martensite, we can think of these variants as deformations of a basic structure,” Liarte said. “In the case of martensite, each cell deforms along one of three directions: length, width or height. Each deformation defines a variant. The different variants combine according to a minimum-energy principle subject to the surrounding conditions.”
However, there is an important difference that makes the study of smectics far more challenging than the study of martensites: in martensites, low-energy configurations can be described as simple 3D rotations of the crystalline variants, whereas in smectics low energy cofigurations may also be produced by other types of transformation. It was on this topic that Liarte and colleagues made their most interesting contribution, using the Lorentz transformation to explain the transition from one variant to another.
Established by Dutch physicist Hendrik Lorentz (1853-1928), the Lorentz transformation is a set of equations that describes how measurements of space and time change when performed in different inertial reference systems. Used later by Einstein, these equations were the mathematical framework for his theory of special relativity, published in 1905.
“One of our collaborators, Randall Kamien from the University of Pennsylvania, recently deduced that the different sets of layers in a smectic could be related to each other by the same equations as those of special relativity, provided the variable time (t) in the Lorentz transformation was replaced by a magnitude that counts the number of layers in the liquid crystal,” Liarte said. “These equations can be used to describe changes in eccentricity between different conics, for example.”
To describe all possible variants, the researchers used four types of transformation: rotation, translation, dilation, and the Lorentz transformation. These four types of transformation comprise Weyl-Poincaré invariance, which contains all forms of symmetry in special relativity.
Republish
The Agency FAPESP licenses news via Creative Commons (CC-BY-NC-ND) so that they can be republished free of charge and in a simple way by other digital or printed vehicles. Agência FAPESP must be credited as the source of the content being republished and the name of the reporter (if any) must be attributed. Using the HMTL button below allows compliance with these rules, detailed in Digital Republishing Policy FAPESP.





